
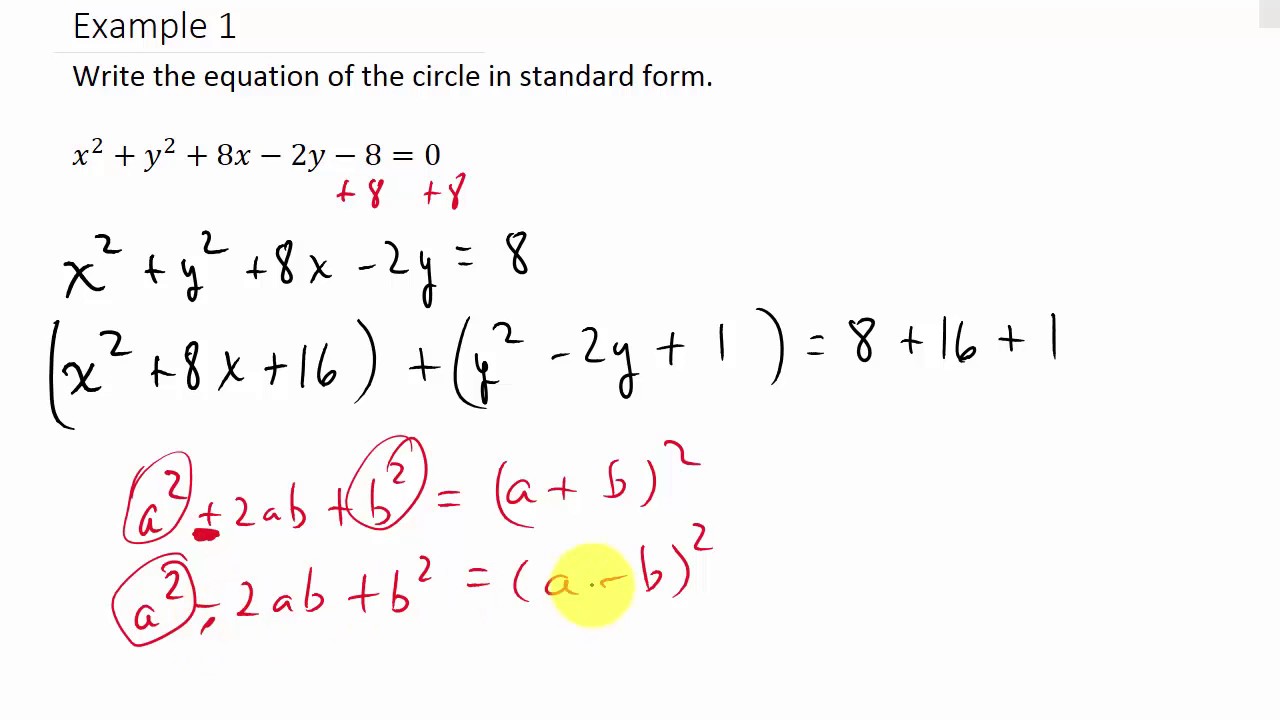
We rewrite our equation to get: \(x^2 - 6x + y^2 + 8y - 1 = 0\)Ĥ) Complete the square for the x and y-terms SEPARATELY. We now have an equation that looks like this: \(x^2 + y^2 - 6x + 8y - 1 = 0\) How? By dividing EACH term in the equation by 4. The magic number comes from treating the x-term and y-termĮxample: Complete the square given the equation \(4x^2 + 4y^2 - 24x + 32y - 4 = 0\)ġ) Do the squared terms have matching coefficients?Ģ) Can we make the squared terms have a coefficient of 1? In this case it will help us get the equation into a more useable form. Remember, of course, that we can always add something to both sides of an equation without unbalancing it. In order to factor the original equation, we will need to add a "magic number" to BOTH sides of the equation. OUR GOAL: To find the standard form of the given circle equation by factoring. We can use a technique called completing the square to rewrite such an equation so that we can quickly identify the circle's center point (h,k) and the radius. See it? If the squared terms have different coefficients, the graph won't be a circle. Notice that the square terms have matching coefficients (A). In general, any equation of the form \(Ax^2 + Ay^2 + Bx + Cy + D = 0\) will produce a circle. Completing the Square Completing the Square (In Circle Equations)


 0 kommentar(er)
0 kommentar(er)
